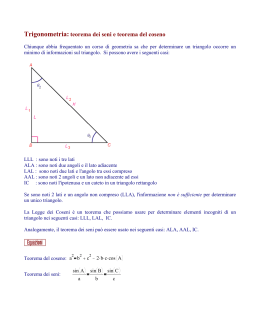
• Rectification on YZ plane
Rectification on XZ plane
• Rectification on XY plane
Detail of the rectified image on XY
plane
ONE (OR TWO) VANISHING POINTS GEOMETRY
The original image (Volubilis, Morok)
RECTIFICATION
Observation of two families of parallel lines
RECTIFIED IMAGE
The deformation on the
upper line are due to lack of
planarity of the mosaic
CORRECT SCALING
• The Vanishing Point Geometry is a powerful tool.
+
• With one Vanishing Point only: the image can be rectified: the
ratio base/height is not correct
• With two Vanishing Points (one horizontal, one vertical): the
image can be rectified: the ratio base/height is not correct
• With three Vanishing Points: the image can be rectified, the ratio
base/height is correct, the orientation can be assessed, both for the
original and the rectified image, apart from one unknown scale
factor
-
• Camera axis must be very inclined
• Only WA are suitable
8) PhotoModeler
by Eos Systems - Canada
• A project:
from the pictures to the recostruction
of the 3-D model
• The inverse camera :
Determination camera parameters
Low-cost software 1000 $
Project:
Options
Selection measure unity
Type of camera
List
resolution of the images
camera parameters
Image Selection in the project
Options of the computation
Punti omologhi collimati in numero minimo
final model with texture
Modello Wireframe
The inverse camera :
Unknown camera parameters
constraints to set
Format .cam
Processo
vincolato
Tests
Sintetic image
given
= -20°
= 40°
Zc= 3.25
0.906 0.088
 0
0.978

T
0.423  0.188

0
 0
0.127 
0  0.064
0  0.273

0
1 
0
B
C 
 
C '
 
 B'
 A
 
D
 D '
 
 A' 
0
1

1

0
0

1
1

0
0
0
1
1
0
0
1
1
1
1
1
1
0
0
0
0
1  0.439
1  0.856
1  1.195
 
1  0.589

1  0.000
 
1  1.022
1  1.302
 
1  0.000
 0.776
 1.181
 0.116
0.279
0.000
 0.239
0.757
0.955
0
0
0
0
0
0
0
0
1
1
1

1
1

1
1

1
Dalla deviazione standard (SQM) si è dedotto che nelle
collimazioni si ha un’incertezza di 0,6 pixel per unità di misura.
PhotoModeer
Dalla deviazione standard (SQM) si è dedotto che nelle
collimazioni si ha un’incertezza di 0,5 pixel per unità di misura.
P
F
3
Cubo 100_30_f28
Results:
Φ
29.935 deg
θ
9.953 deg
10 deg
Κ
-0.010 deg
0 deg
f
27.957 mm
28 mm
ppx
400.546 pxl
400 pxl
ppy
299.985 pxl
300 pxl
Expected:
30 deg
P
F
3
Cubo 60_15_f35
Results:
Φ
15.453 deg
θ
-30.038 deg
-30 deg
Κ
-0.245 deg
0 deg
f
35.001 mm
35 mm
ppx
394.151 pxl
400 pxl
ppy
297.443 pxl
300 pxl
Expected:
15 deg
Ancona – Traiano’s arch – Model formation by Photomodeler
Ancona – Traiano’s arch – The residuals of the Model formation
Ancona – Traiano’s arch – the camera stations
A practical example of 3Dreconstruction
The Ho Chi Minh’s house in
Hanoi
Back sie
Right side
Left side
Front
inverse Camera
Interior camera
Parameters stored
formato .cam
Some phases of the observation and referencing process
Points in 3D space
Height of the
parapet =
1 Module
PF3 measurements
Interior
Exterior
PhotoModeler
P
F
3
Model Recostruction in AutoCad
PhotoModeler:
utilized
by non-specialised operators
very
powerful
Very
good interface
Many
options
3D

modeling
VRML export
Rectification
Non-metric
Control
No
Only
images
informations
need to supply approximate value
one control information is missing: the camera station
coordinates
9)
• ARPENTEUR
by Pierre Drap – Pierre Grussenmeyer
• (ARchitectural PhotogrammEtry Network Tool for EdUcation and
Research).
• Designed in 1998 by two research teams (GAMSAU-CNRS and
ENSAIS-LERGEC)
• Mainly for Education
• The running from the net
• Thus it can be easily and freely used
– from anywhere, by anybody all over the world and
– with whatever operating system.
• www.arpenteur.net.
• Dedicated to architectural photogrammetry and
close range terrestrial photogrammetry, but
aerial images (limited to a few Mb) can be also
handled.
• Photos from a wide range of calibrated cameras
– metric
– non-metric camera,
– digital amateur camera
• ARPENTEUR permits a better knowledge of basic
photogrammetric techniques
•
•
•
•
stereoplotting,
image correlation,
architectural photogrammetry
architectural modelling
• Output results can then be viewed as text-file, DGN,
DXF, VRML file for a further processing with
Internet or CAD systems (e.g.: MicroStation,
AutoCad).
Conclusions:
• There are today on the market many good
products for any purpose and for any pocket
• The quality of a system is based not only in the
accuracy but anso in the efficiency
• Photointerpretation
• Orthophoto
Acknowledgements
Thanks to my students who helped me, part of this material is
their graduation thesis:
• Matteo Cinti, Marco Battistelli
• Paolo Clini
• Gianluca Gagliardini
• Stefano Benassi
• Paolo Margione
• Loretta Alessandroni
• Ivan Catini
• Floriano Capponi
• Ingrid Luciani