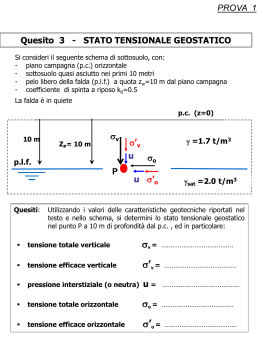
Circuito RLC-serie forzato
V (t)=V0 cos(w t) = Re(V0 eiwt )
V0 =10 V
w =5 p rad/s
R=2
L= 0.15 H
C=0.05 F
|Z|=
R 2 + (w L -1 / wC)2 = 2.74W
f = arctan[(w L -1 / wC) / R] = 0.82rad
æ V0 eiwt ö
æ V0 iwt-f ö
V0
I(t)=
cos(w t - f ) = Re ç
= Re ç
e
÷
iwf ÷
è
ø
|Z |
|
Z
|
e
|
Z
|
è
ø
Rappresentazione nel piano complesso
(metodo simbolico o dei fasori)
Complesso!!
I
m
~V(t)
f
V0
~I(t)
wt
Re
Complesso!!
Andamento ondulatorio
descritto dalla proiezione
lungo l’asse Re
Rappresentazione reale in funzione dell’angolo wt
10
5
0
-5
- 10
0
0.1
0.2
V(t)=V0 cos(w t)
0.3
0.4
0.6
V0
cos(w t - f )
|Z |
0.5
I(t)=
0.7
0.8
0.9
1
wt
f
10
5
0
-5
- 10
0
Corrente:
• Ampiezza minore rispetto a tensione
• Stessa pulsazione w (periodo T, frequenza n, lunghezza d’onda l)
• Fase differente: in questo esempio la corrente è in RITARDO rispetto alla tensione
0.1
0.2
0.3
Rappresentazione nel piano complesso
V(t) = V0eiwt
Im
~V(t)
Z = R + i(w L -1 / wC)
|Z|=
R + (w L -1/ wC)
2
2
f = arctan R / (w L -1 / wC)2
V(t) V0 eiwt V0 iwt-f
I(t) =
=
=
e
if
Z
|Z |e
|Z|
f
~VC(t)
~VR(t)
~I(t)
wt
~VL(t)
Re
10
5
0
-5
- 10
0
10
Rappresentazione reale in funzione dell’angolo wt
Corrente e Tensione su Resistenza
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
0.9
1
wt
wt
8
6
4
VR (t) = I 0 Rcos(wt - f ) in fase con I(t)
2
0
-2
-4
-6
-8
0
6
I(t)=I0 cos(wt - f )
0.1
0.2
0.3
0.9
0.9
wt
0.1
0.1
0.2
0.2
0.3
0.3
0.4
0.4
0.5
0.5
0.6
0.6
0.7
0.7
0.8
0.8
0.9
0.9
1
1
wt
VL (t) = I 0w L cos(wt - f - p / 2) = I 0w Lsin(w t - f )
I(t) in ritardo di p /2 su VL (t)
-6
-8
0
6
4
2
0
-2
-4
-6
0
p/2
I(t)=I0 cos(wt - f )
1
1
Rappresentazione reale in funzione dell’angolo wt
Corrente e Tensione su Induttanza
.9
wt
1
8
6
4
2
0
-2
-4
-6
-8
0
6
Rappresentazione reale in funzione dell’angolo wt
Corrente e Tensione su Condensatore
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
0.9
1
p/2
I(t)=I0 cos(wt - f )
VC (t) =
wt
I0
I
cos(wt - f + p / 2) = - 0 sin(wt - f )
wC
wC
I(t) in anticipo di p /2 su VC (t)